How Do You Determine Which Note In An Interval Is The Root Note?
If I played any two notes together, which one of them would be considered the root note?
Whatever the lowest note is?
Neither of them?
Both of them?
Actually... none of these are the correct answer!
The correct answer is something that you would never think of in a million years. It’s so shocking that I would recommend you sit down before reading what the real answer is.
Are you sitting down? Alright, here we go.
The correct answer of which note of a given interval is the root note is...
It depends
Shocking, I know. So non-committal, so recklessly ambiguous, so frustratingly uncertain.
What does it depend on? Dare you even ask?
It depends on everything, and yet nothing at the same time. It depends on the sun and the moon, the planets and the stars. It depends on blue, it depends on green, and on all the colours in between. From pleasant views of land and sea, it depends on you, and it depends on me.
No, no, it just depends on the interval. All the details and the rationale behind it are awaiting you in the video below:
Speaking of intervals and root notes, these are both very big components of understanding chords and harmony on the guitar - just like the video mentioned! If you want to understand chords better, check out my Complete Chord Mastery guitar course.
Video Transcription
Hello Internet, so nice to see you! we know that chords have roots, we know that you play a C major chord. The C is the root of this chord. But do intervals have roots? If I play only two notes? Can I legitimately call one of those notes the root? And if so, which one? Well, it turns out that yes, intervals do have roots, it's actually pretty well known if you study music theory in depth, okay? And it's quite obvious which one it is okay.
And indeed, I'm gonna explain how and why that basically, I'm gonna say it immediately, there is a very, very simple criterion, which I'm going to reveal in a second, because before of that, I have to tell you about the story of how I learned all of that I was reading a music theory book, and his music theory book, I'm not gonna say the name of this book, because honestly, I know half of you, we're gonna go and buy this book. And you'll see in a moment why you shouldn't do that. Okay? Because in this book, the first 80 pages were about how to build a major scale, starting from first principle, I know like temperaments, frequencies and stuff.
And then other 80 pages were about on finding a scientific theory on how to find the interval, the root of every interval. And the guy spent at eight zero pages. We explain in this theory, using the undertones, which I should remember, everybody undertones do not really exist in physical reality, they're an obstruction. But yeah, he's speaking about undertones and stuff. And he goes on, and it takes forever.
And it applies this theory to all the 12 possible intervals, and defines that his theory gives the right answer in six out of the 12 intervals, and on the other six, it gives the wrong answer. And given that you have only two choices, I mean, in two unknowns, it's one or the other being this theory, that takes you 80 pages of technical language to learn as exactly the same accuracy as a coin toss. Wow.
That's why I never recommend books or music theory, because they are all like that. Hmm. Anyway, what is the root of an interval? It's simple, the root of an interval is just the root of the simplest chord, you can fit to that interval. Let me explain. Let's see, for simplicity, that your interval, it's a major third, and I'm going to take the note C, and the note E. Okay, so immediately, you can see that you can fit a C major chord to it, C, E, G, is just so the C and E, we can pretend for a moment is just the C major chord in which I eliminated the G.
And if this is the case, C is the root because C is the root of the chord. And so it's going to be the root of the interval. Of course, at this point, all of you are protesting raising your hand banging your fist on the table, like, oh, man, this is preposterous. I can fit another chord in that yes, definitely, you can even fit, for instance, an A minor chord to this, I mean, wouldn't be bad, right?
You can fit an A minor chord to it, the notes of the A minor are a C and E. And so the root of the interval c and e will be oh wait will be the A and the A it's not in the original interval. So the A cannot be the root of C and E. Because it's neither C or E, right? There are many other intervals, you can fit to that you can even fit an F major seven with the notes, or F, A, C and E. But again, the C and E are the fifth and the seventh of this chord and the root is F and I cannot say that f is the root of the major third C and E. But of course, this still doesn't demonstrate anything.
Some of you can say, well, tomorrow, I can take an E minor with a flat six, the notes are going to be E, G, B, and C. And the root of these chord is E. Well, yes, you could do that. You could also notice incidentally, that these chord has exactly the same note as a C major seven chord and the root of the C major seven is definitely C not E. So still, but that's the other thing. You had to fit a very dissonant interval here. Okay, in the chord because on the E minor prior, this flat six is pretty decent.
I mean, it sounds this way. Ouch. Okay, so you need really need to use a complex chord in a sense and see the notation is more complex. What's more complex, E minor, flat six, or C major. Right? So you see that C major is the simplest chord you can fit to that. And so C is the root of this interval. And we can do this for every interval. Indeed. Let's pick all the interval if the interval is a major third or a minor third, the A root of the interval is always going to be the lowest note because you can simply fit a major triad or a minor triad, just like I did for C and E for the inversion of those intervals.
So if I have a major sixth or a minor sixth, then well, the notes are still the same notes, right, and just inverting the interval, it makes no difference if I have a C, and an E, or an E and A C, which means that for the six, the root note of this interval is the top note not the bottom. So for e to see the truth, it's C. And if I had a major six, like E flat C, the top note C will still be the root.
Yay, what if I have a fifth or a fourth? Well, if I have a fifth, let's pick C and G. Well, you see, again, the simplest chord, I can fit to these it's either a C major triad or a C minor triad, I mean, if I have C, and G, either I play C, E, G, or C, E flat G. But either way, the root is still C. So for a perfect fifth, the root, it's the bottom note. If it was a perfect fourth, which is the inversion, there's also going to be the top note because again, it's G and C, for instance. And again, I can still fit a C major or C minor prior to it.
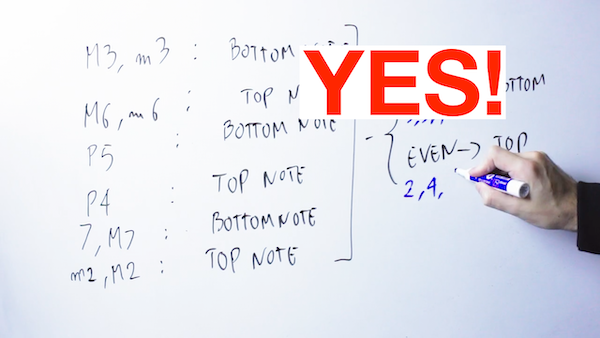
It's just a matter of trying fit trying, and fitting the simplest chord you can find again, and so it requires you to guys to be familiar with the chords. But again, the rules are all here and writing them down right now, for a third, it's always the bottom note. For a sixth, it's always a top note for a fifth, it's the bottom note. For fourths, it's the top note and they're gonna say, I'm going to summarize that them really easily in a second.
Okay, what about sevens? Well, I can have major sevens or minor seventh. So let's see, I have I didn't know, minor seven. So let's say G to F, I'm going to make it easy. I can fit several chords to these. And if I go from G to F, I can fit dominant seven to eight, or a minor seven. Okay, for instance, I can even fit minor seven, with a flat five. But all those chords have the root, G, okay, and every other chord they can fit that will not have either g or f as the root. So on a seven is the bottom note.
And this is going to be through the same for major sevens. Meaning if I have something like C and B, so C at the bottom, B at the top is a major seventh I can fit here as C major seven, and the root, it's C. So for the seventh chord, it's still the bottom note, and the second inversion of the seventh. So for the second, the top note is the root. If I play a C and the D node, the D is the root because I can fit these to the seventh or D minor seven.
So far, so good, guys, all of these seems a little bit complex, okay, but it's not so much because at the end of the day, you'll notice immediately, if the number we use to indicate the interval, it's odd 357. Then the bottom note is the root. If the number we use to indicate the interval, it's even 246 is the top note that is the root boom, simple as that odd number, bottom note, even number top note.
Now why will you need to know all these simple, because sometimes you want to express a chord. And you want this chord to be understood, but you want to use only two notes. So it's important to know which one is the root so you don't play the wrong two notes and came up with something different.
Okay, if you want to express a C major chord and you want to use only two notes, you need to play either C and E, so the root and the third, or C and G, the root and the fifth, don't you play C and G remember, it could be ambiguous, it could also be a C minor. But if you play that it's clear if you play only those two notes, and nobody else is playing any other note and only those two notes are heard, at least the listener can understand that the root note is C because for some reason, that's what your ear does, it hears two notes and marks one of them as being the most important the root and the other one to be an auxiliary, the other note, okay, and understands chord progressions this way.
And using this trick, you can write any chord progression using seven chords, minor chords, major chords, etc cetera just by picking one interval out of them and one note is the root and one is notice another note of the chord. And if you pick them the right way, you're always going to have that it's clear which one is the root. Okay, simply take a chord progression, any chord progressions, that eliminating note, apply these and you'll see how to do it Okay, sometimes you really desire To have this kind of ratified texture where you use only two notes and in this case, you need to know which of those two notes is the root so that you know what is going on.
Now if you want to know more about how to manipulate the texture of your chord progression, so that you can use less note to imply more complex chords, or you can change how the very same chord sound by playing them in a different way on your guitar. Well that takes a little bit more time to learn. And if you want to do that, I will recommend you guys have a look at my course complete chord mastery, complete chord mastery.
It's not a book. It's a complete video course that takes you from the basics up. We do everything you need to know about harmony and chords on your guitar. All the theory is done straight on the fretboard. There is no theory for the sake of theory here. Everything is immediately practical. And everything is developed through exercises so you know how to apply these immediately on your guitar. If you have just a minute click on the link on the top right to check out complete chord mastery.
If you liked this video, smash that like button and don't forget to subscribe and click on notification otherwise YouTube will not let you know when I put up a new video. And if you have any comments, feedback, suggestions, write them down in the comment. I enjoy reading from you and they make videos on your suggestions. This is Tommaso Zillio for musictheoryforguitar.com, and until next time, enjoy.